Culturally Relational Education In and With an Indigenous Community
Dwayne Donald, Florence Glanfield, and Gladys Sterenberg
University of Alberta
Aboriginal Student Performance in Mathematics
In Canada, postsecondary enrolment and completion rates of Aboriginal students are significantly lower than those of non-Aboriginal students (Canadian Millennium Scholarship Foundation, 2004; Statistics Canada, 1996). This is most evident in disciplines involving science and mathematics (Indian and Northern Affairs Canada, 2005). Furthermore, Aboriginal student achievement in K–Grade 12 mathematics courses is significantly lower than those of non-Aboriginal students (Neel, 2007).
As researchers, we have noticed that there is a sense of urgency to learn about ways in which Aboriginal children can be more successful in mathematics education. Communities are looking for solutions. Several research projects engage communities in solution-finding projects. For example, there are projects that explore the ways in which the experiences of Aboriginal communities might be used to explore mathematical concepts (e.g. Lipka, 1994; Lipka et al., 2005). Further, there are suggestions that Aboriginal peoples are regularly engaged in mathematical activities (e.g., in construction of snowshoes & teepees; Native Access to Engineering, n.d.), that mathematics exists within cultural artifacts (e.g., pattern-making in beading; Eglash, 2003), and that careful attention to the unique learning styles of Aboriginal students will inform and enable more culturally-appropriate teaching (Boulton-Lewis, Marton, & Wilss, 2001; Cooper, Baturo, Warren, & Doig, 2004; Ryan, 1992).
Within such a context of urgency, we were invited by Eagle Flight First Nation, located in what is now called the province of Alberta, to provide leadership and to conduct research in order to improve student performance on the Provincial Achievement Tests in mathematics. As outside experts, we could have been expected to respond to this invitation by imposing on school staff (teachers and assistants) those instructional tools and strategies we believed would result in increased student achievement at Eagle Flight School. However, we interrupted these deficit stances by listening to Samuel (the band councillor responsible for education), his father Stanley (former chief and band councillor), the staff, and the children. Our research has emerged in the moments of dialogical interaction, not in unidirectional responses. The unfolding of our work with Eagle Flight First Nation prompts us to deeply reflect on what it means to “do research” in and with an Indigenous community and on the meaning of culturally relational education.
When invited to participate in this work with Eagle Flight First Nation there were many different stances that we might have taken in our response. In the next sections, we will describe the different responses we considered and then share with you the response that emerged and continues to unfold in our research in and with Eagle Flight First Nation.
Stance 1: A Mathematically Deficit Response
One stance that we could have taken when we were first invited to work with Eagle Flight First Nation was to focus the research on the so-called achievement gap and the mathematical knowledge needed by both students and teachers to bridge this gap. In our view, the difficulty with this stance is that an authoritative status of mathematics is asserted and we are situated in roles as outside experts who will provide “the way” to success. We could have said to Eagle Flight First Nation, “If you adopt program X that has proven to be successful in another location, then achievement in mathematics in your school will improve.” Swetz (2009) problematizes the adoption of program models by drawing on the results of the 2003 Trends in International Mathematics and Science Study (TIMSS): “As a result of this assessment, several countries are now attempting to emulate Singapore by adopting its curricula and teaching methods. Beware!” (p. 36).
Swetz (2009) also provides a second example of this authoritative status. Swetz suggests that mathematics programs are often adopted because of the mathematical authority of the curriculum developers. So, our response to Eagle Flight First Nation could have been, “Adopt Program Y because the authors of the program are experts in mathematics.” Swetz (2009) acknowledges the challenges inherent in implementing a program that relies on authoritative mathematical knowledge by describing the African government's adoption of a mathematics program created by Zacharias, a physics professor from Massachusetts Institute of Technology (MIT):
It seems that in the bland adoption of Western experimental curriculum into an African situation, no consideration was given to the cultural and socioeconomic impact. When USAID [United States Agency for International Development] officials were questioned on Jerrold Zacharias’s experience and expertise involving education in poor, developing, countries, they responded that his association with MIT was sufficient. Apparently, the aura of MIT’s excellence in science and mathematics was omnipotent! This was an example of educational imperialism at its worst! Available research findings on mathematical teaching and learning in the developing world were ignored. (pp. 34-35)
Swetz suggests that mathematical programs of study cannot be imported across cultural boundaries without considering the pedagogical implications and socioeconomic complications of such an adoption.
We encountered a similarly related assumption related to Provincial Achievement Tests and the provincial program of studies. Given that the content of the Provincial Achievement Tests is based on the learning outcomes described in the provincial mathematics program of studies, one way to close the achievement gap in mathematics is through adherence to programs of study that reflect mathematical standards and systemic structures that promote accountability through high stake testing. Our own experiences with programs of studies across North America (e.g. Alberta Education, 2007; National Council of Teachers of Mathematics, 2000) prompts us to question the explicit emphasis on goals, standards, expectations, general and specific outcomes, and achievement. The authority of such programs is shown through the arrangement of mathematical concepts into a spiral curriculum of four organized strands and suggests that student learning can be predetermined. Knowledge is viewed as a commodity where students develop a “base of knowledge” and they are expected to experience the “power and usefulness of mathematics” (Alberta Learning, 1997, p. 2). The content is stated as outcomes that are “measurable and identify what students are expected to know and do” (Alberta Learning, 1997, p. 13). Conformity to social, political, and economic goals is pursued. Schooling is functional as students are prepared for productive lives in a “rapidly advancing, technological society” (Alberta Learning, 1997, p. 2). The authoritative position of mathematics in society is rationalized since proficiency in using mathematics “increases the opportunities available to individuals” (Alberta Learning, 1997, p. 2). Rather than seeking to change existing social structures, this program of studies reproduces social inequities by supporting an underlying ideology of authoritativeness as “all students should receive a level of mathematics education appropriate to their needs and abilities” (Alberta Learning, 1997, p. 2). The assumption that mathematics is authoritative implies that improving student achievement in mathematics might include imitating best practices of model programs, schools, and teachers who have experienced academic success (often interpreted as success on standardized tests) in mathematics.
Further, in our review of programs of studies across North America, we noticed that these provincial (or state) defined programs reflect colonial logics where people with mathematical authority set the priorities. The underlying assumption of a mathematically deficit stance is that not all students are capable of learning mathematics and an authoritative person must decide on students' needs and abilities in order to implement the program at the students' levels. A focus on best practices suggests a unilateral predetermination of what is needed in all learning contexts and emphasizes a notion of mathematics as universal and culture-free. Mathematics appears to be universal because of the prevalence of absolutist philosophies that view mathematics as timeless because it builds on logics of deduction. In this perspective, mathematical knowledge is
superhuman and ahistorical, for the history of mathematics is irrelevant to the nature and justification of mathematical knowledge; it is pure, isolated knowledge, which happens to be useful because of its universal validity; it is value-free and culture-free for the same reason. (Ernest, 1994, p. 339)
Mathematics is viewed as culture-free because "every trace of human effort and activity (and value-ladenness) is expunged from the final printed version" (Ernest, 1994, p. 52). Moreover, by ignoring the historical development of mathematical knowledge in non-Greek places such as Africa, China, Middle East, India, Central and South America, the myth of mathematics as a European discipline is promoted and sustained. Colonial logics underlie this perspective as the European project of domination continues.
While we
understood that we were invited to work with Eagle Flight First
Nation because we were positioned as mathematics education and
curriculum experts, we resisted the authority of this role and
wondered, where do the experiences of the individuals who are
expected to
“meet these standards” and those who are
expected to “teach to these standards” fit in the
mathematics as authority discourse found in programs of study?
Stance 2: A Culturally Deficit Stance
Often the so-called achievement gaps are interpreted according to a cultural-deficit model (Salkind & Rasmussen, 2008). When considering this stance, one assumption is that Aboriginal communities do not have the ability to actively support the academic achievement of their children. In an effort to help formerly colonized peoples heal and revitalize their communities through the identification, teaching, and learning of relevant notions of culture, Indigenous education initiatives around the world have promoted culture-based curriculum as a restorative tool.
In the North American context, culture-based curricular approaches are similarly viewed by many educators as the key to changing the educational experiences of Indigenous peoples (Aikenhead, 2002; Au & Jordan, 1981; Battiste, 2000, 2002; Castagno & Brayboy, 2008; Ezeife, 2002; Gay, 2000; George, 2003; Ladson-Billings, 1994, 2009; Lipka & Stairs, 1994). Such calls for culturally responsive education respond to the difficult experiences that Indigenous peoples have had with formal schooling. In general, the colonialist solution to the perceived problem of bringing education to the Natives was to follow a coercive and abusive program of assimilation during which Indigenous children were subjected to a forced forgetting of the languages and knowledges learned from their families and communities. Residential school policies forcefully removed Aboriginal children from their homes, housed them in harsh environments, unravelled their connections to their cultural values, identities, families, languages, and spiritual practices, and disrupted the functioning of family and cultural institutions (Miller, 1996; Milloy, 1999). At its height in Canada, the residential school program was an all-encompassing experiment in the resocialization and civilization of Indian children. The response to develop culturally responsive education offers a way to disrupt colonial logics.
While research on culturally responsive education is insightful, it has had little impact on what teachers do because, we believe, it is too easily reduced to essentializations, meaningless generalizations, or trivial anecdotes—none of which result in systemic, institutional, or lasting changes to schools serving Indigenous children (Castagno & Brayboy, 2008). St. Denis (2004) confronts this problem by suggesting that the focus on cultural revitalization in Aboriginal education in the wake of the residential school experience has led to the predominance of a vexing form of cultural fundamentalism in the field: “Adherence to cultural revitalization encourages the valorization of cultural authenticity and cultural purity among Aboriginal people and has helped to produce the notion and the structure of a cultural hierarchy. ‘Authentic’ cultural Aboriginal identity has become high currency” (p. 37). She argues that cultural fundamentalism has created a particular problem for many Aboriginal teachers and students who come to feel culturally inadequate as Indians because they cannot perform their culture or language in authoritatively authentic ways. St. Denis contends that the project of cultural revitalization frames Aboriginal peoples as responsible for “losing” their culture and languages; “they are produced as reckless caretakers of their culture” (St. Denis, 2004, p. 43). Importantly, she forwards the view that this emphasis on cultural revitalization in Aboriginal education distracts from and minimizes the historic and ongoing systemic racism and discrimination that has also so deeply affected Aboriginal peoples:
The popular notion that one has lost one’s culture, as opposed to having one’s culture stolen, places the responsibility for making appropriate cultural adjustments on those who for so long were the target of systemic and individual cultural change…Describing Aboriginal youth as lost is a benign way to describe the effects of the discrimination, exclusion and sustained violence and aggression they face on a daily basis. (St. Denis, 2004, p. 43)
If we reject a mathematically deficit stance and culturally deficit stance as neither viable nor generative, then another consideration might involve a culturally relational stance. We have come to think of our practice in this way. This means that we are all related: We are relations.
Stance 3: A Culturally Relational Stance
We were invited to engage in research in the community (as represented by the band councillors) and we invited the community to engage in research with us. When we first met the school staff, we invited them to be co-researchers with us and the community in this research. Together with the community and school staff, we worked to define our community-based research project. Our research project explores the ways in which community members, children in school, school staff, and school and community leadership come to develop a shared understanding of mathematics. The first stage of this project, defined after a year of meeting with the community members, teachers, and staff, has been to develop an understanding of the ways in which children in the community know mathematics. We are in the fourth year of the first stage of this project and children and teachers in Grades K-9 are participating. The teachers identified the need to know what their children could do mathematically so, together with the teachers, we designed a variety of assessment strategies such as performance-based tasks and interviews to develop an understanding of the way in which the children in their classrooms think about mathematical ideas. In the second year of the project, we worked alongside three staff-identified lead teachers as we engaged children in mathematical interviews and collected data on children’s thinking through video-recordings. The recordings were analyzed by this team of teacher/researchers and then we shared video clips and preliminary results with the rest of the school staff. In the third year of the project, the teachers of the entire school decided to engage their children in paper-and-pencil and performance task assessments. These assessments were designed by the researchers and the initial lead teachers, and then administered by all teachers. The results were analyzed by all staff during a multi-day in-service meeting at the beginning of the fourth year.
Another aspect of the project is mathematics teacher development. In this fourth year, teachers are using what they are learning about children in their classes to inform planning and classroom practices. Specifically, they are focusing on mathematical vocabulary development and teaching mathematics with manipulatives. Assessments of children’s thinking are ongoing and data collected on these assessments is shared with us. During staff in-service meetings, our conversations about what teachers are learning from children when children are asked to explain their mathematical thinking are recorded as data. Insights into the ways in which teachers are learning inform our ongoing work together.
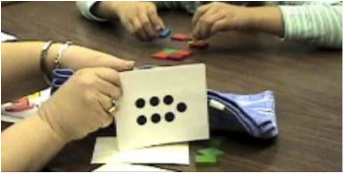
An excerpt of one initial interview shared with the staff provides a glimpse into our relational practice. This interview is part of the ongoing study investigating the ways in which children come to know mathematics. Amelia, a Grade 2 student, is being interviewed. Two researchers are present and Amelia’s teacher has been invited to participate as an observer and as an additional interviewer (as a part of the mathematics teacher development).
Florence: “I’m going to show you some dots on a card. Amelia, I want you to look at the number of dots and I’m going to show them for just a short period of time and I want you to tell me how many dots there are on the card. Ready?”
Amelia [Amelia rests her chin on her hands]: “Seven?”
Florence: “How did you see the seven?”
Amelia: “I don’t know cause.” [Amelia points to her forehead with both hands.]
Florence: “You counted with your fingers?”
Amelia: “In my head.”
Florence: “In your head? So if I showed you the card, could you tell me how you saw, how you counted it?” [Florence places the card on table.]
Amelia: “I just saw three and one more is seven.” [Amelia moves her finger over the two rows of three].
Florence: “Ah… So…”
Amelia: “Three plus three is six and I just added one more. Seven.” [Amelia is placing tiles in a line.]
Florence: “Wow. Thank you. You explain those so well.”(transcribed video recording, December 8, 2008 )
As researchers, we noticed the mathematical robustness of Amelia’s thinking. She was able to recognize parts of a whole, she was proficient in subitizing (meaning she was quickly able to recognize small quantities), and she was confident in providing an explanation of her thinking. We also noted that rather than simply observing Amelia, Florence sought to relate to the student. She invited Amelia’s teacher to sit alongside Amelia and to participate as an observer and interviewer in order to put Amelia at ease. Florence’s role was to invite Amelia into a reciprocal relationship and Amelia seemed very willing to engage in this way with Florence as the interview continued. It is important to note that Florence’s role was not that of a tester or examiner where she is looking for the correctness of Amelia’s responses. The purpose of the interview was to better understand Amelia’s reasoning. Florence’s responses to Amelia’s explanations were open and encouraging. Yet further in the interview, there is evidence that prompts were required when Florence did not grasp Amelia’s reasoning. Rather than rephrasing the question or providing an alternative question, Florence provided Amelia with the opportunity to demonstrate her reasoning in a variety of ways by using tiles, drawings, and descriptions.
Amelia’s teacher was also impressed with Amelia’s mathematical understanding as she listened to Amelia’s explanations and observed Amelia’s actions. Describing this student as someone who hates worksheets, the teacher was profoundly impacted:
She just flourished in that kind of testing environment and I was really surprised at how smart she really was. She was showing me a whole different level of her thinking that I didn’t even know she could do those kind of problem-solving tasks. So I was really – that was a real eye-opener for me for sure. Now I’m going to start assessing them a little bit differently and use some of those testing manipulatives that we were using yesterday. (Amelia’s teacher, transcribed audio recording, December 8, 2008)
In this particular situation, we (the authors and Amelia’s teacher) are learning from a child. In the context of this research, we are relations of the students, their families, the school staff, the children, and the band councillors. In a sense the three of us are relations of the individuals who work at Eagle Flight School and we are relations of the whole community. We wonder, what does it mean to be a relation and to work firmly in a relational context? Another example taken from field notes written by Florence might provide insight:
Seven-year-old Amelia and her teacher knock at the door and Amelia bounces into the room, smiling and ready to be video-recorded. Amelia is dressed up in a deep purple shirt and her hair is styled for this second mathematics interview. At the end of the interview, Amelia reminds us that we were supposed to send her a recording of the first interview and that she and her family, including her kookum, have been waiting to see it. In this moment, the three of us look at one another and realize that we are in a relationship with Amelia, her family, and her school. (Florence, field notes, May 28, 2009)
Through our listening to the teachings of children and school staff, we have come to conceptualize culturally relational education from an Indigenist research paradigm. Wilson (2007) uses the term Indigenist to label a research paradigm related to Indigenous perspectives. He chooses to use this term because he believes “that an Indigenist paradigm can be used by anyone who chooses to follow its tenets” (p. 193). Wilson (2007) also suggests that in order to describe and use an Indigenist research paradigm,
researchers and authors need to place themselves and their work firmly in a relational context. We cannot be separated from our work, nor should our writing be separated from ourselves (i.e. we must write in the first person rather than the third). Our own relationships with our environment, families, ancestors, ideas, and the cosmos around us shape who we are and how we will conduct our research. Good Indigenist research begins by describing and building on these relationships. (p. 194)
Throughout our research, we live with the messiness of being in relation. The research data is collected by members of the school community and is shared with us. We work with one another, and with the community and school staff, to develop an understanding of the ways in which the data might be used. Significantly, we are with the community in multiple ways. We work alongside one another and with the community and school staff to question the ways in which the notion of culture is typically taken up. In this work, alongside the community and school staff, we challenge our assumptions about what the culture says the students should be and we are coming to understand and honour the philosophies that underlie the culture of the community. This is what the term culturally relational has come to mean to us.
Conclusion: Miyo-Wichitowin
Our consideration of a culturally relational stance is informed by what we have learned about the value of listening. Listening is critical in being relations. The Blackfoot concepts of aoksisawaato’p (visiting/renewal of relations), aokakiosiit (be wisely aware; pay attention), and aatsimaak’ssin (responsibility to balance giving/taking reciprocity) inform our practice as culturally relational. Reconsidering a perspective of the authoritativeness of mathematical knowledge and a culturally deficit stance, we propose an emergent and generative relationality. We suggest that in our research, we bring forth a world of significance where knowing arises in action because of the highly relational nature of learning and social interaction as explicated by enactivists Maturana and Varela (1987): "This circularity, this connection between action and experience, this inseparability between a particular way of being and how the world appears to us, tells us that every act of knowing brings forth a world" (p. 26). Knowing is doing. Knowing is enacted. Knowledge is relational (Davis, 1995; 1996).
Culturally relational education is also profoundly dependent on the Cree concept of miyo-wichitowin, a healing energy or medicine that is generated when we are actively together with the intention of honouring and respecting the relationships we are enmeshed within. As educators and researchers, we came to adopt the stance of being relations in and with Eagle Flight First Nation. As co-researchers alongside the councillors, staff, and children, we are seeking to honour meaningful engagements with Indigenous philosophies and knowledge systems as they are understood and lived by all in relation.
References
Aikenhead, G. (2002). Cross-cultural science teaching: Rekindling traditions for Aboriginal students. Canadian Journal of Science, Mathematics and Technology Education, 2(3), 287-304.
Alberta Education. (2007). The Alberta K-9 mathematics: Program of studies with achievement indicators. Edmonton, AB: Alberta Education.
Alberta Learning. (1997). Mathematics kindergarten to Grade 6. Edmonton, AB: Alberta Learning.
Au, K. H., & Jordan, C. (1981). Teaching reading to Hawaiian children: Finding a culturally appropriate solution. In H. T. Trueba, G. P. Guthrie, & K. H. Au, (Eds.), Culture and the bilingual classroom (pp. 139-152). Rowley, MA: Newbury House Publishers, Inc.
Battiste, M. (2000). Maintaining Aboriginal identity, language, and culture in modern society. In M. Battiste (Ed.). Reclaiming Indigenous voice and vision (pp. 192-208). Vancouver, BC: University of British Columbia Press.
Battiste, M. (2002). Indigenous knowledge and pedagogy in First Nations education: A literature review with recommendations. Ottawa, ON: Indian and Northern Affairs.
Boulton-Lewis, G., Marton, F., Wilss, L. (2001). The lived space of learning: An inquiry into Indigenous Australian university students’ experiences of studying. In R. J. Sternberg & Zhang, L. (Eds.), Perspectives on thinking, learning, and cognitive styles. Mahwah, NJ: Lawrence Erlbaum Associates.
Canada Millennium Scholarship Foundation. (2004). Aboriginal peoples and post-secondary education: What educators have learned. Retrieved from http://www.millenniumscholarships.ca/images/Publications/aboriginal_en.pdf
Castagno, A., & Brayboy, B. (2008). Culturally responsive schooling for Indigenous youth: A review of the literature. Review of Educational Research, 78(4), 941-993.
Cooper, T. J., Baturo, A. R., Warren, E., & Doig, S. M. (2004). Young “White” teachers’ perceptions of mathematics learning of Aboriginal and non-Aboriginal students in remote communities. Proceedings of the 28th Conference of the International Group of the Psychology of Mathematics Education (Vol. 2, pp. 239-246).
Davis, B. (1996). Teaching mathematics: Toward a sound alternative. New York: Garland Publishing.
Davis, B. (1995). Why teach mathematics? Mathematics education and enactivist theory. For the Learning of Mathematics, 15(2), 2-9.
Eglash, R. (2003). Culturally situated design tools: Teaching math and computing through culture. Retrieved from http://csdt.rpi.edu/
Ernest, P. (1994). The philosophy of mathematics and the didactics of mathematics. In R. Biehler, R. W. Scholz, R. Strässer, & B. Winkelmann (Eds.), Didactics of mathematics as a scientific discipline (pp. 335-350). Dordrecht, The Netherlands: Kluwer.
Ezeife, A. N. (2002). Mathematics and culture nexus: The interactions of culture and mathematics in an Aboriginal classroom. International Education Journal, 3(2), 176-187.
Gay, G. (2000). Culturally responsive teaching: Theory, research, & practice. New York: Teachers College Press.
George, P. (2003). The rainbow/holistic approach to Aboriginal literacy. Canadian Journal of Native Education, 27(1), 29-40.
Indian and Northern Affairs Canada. (2005). Aboriginal post-secondary education and labour market outcomes Canada 2001. Retrieved from http://www.ainc-inac.gc.ca/pr/ra/pse/01/01_e.pdf
Ladson-Billings, G. (1994). The dreamkeepers: Successful teachers of African American children (1st ed.). San Francisco, CA: Jossey-Bass Inc., Publishers.
Ladson-Billings, G. (2009). The dreamkeepers: Successful teachers of African American children (2nd ed.). San Francisco, CA: John Wiley & Sons, Inc.
Lipka, J. (1994). Culturally negotiated schooling: Toward a Yup’ik mathematics. Journal of American Indian Education, 33(3), 14-30.
Lipka, J., & Stairs, A. (1994). Negotiating the culture of Indigenous schools [Special issue]. Peabody Journal of Education, 69(2).
Lipka, J., Hogan, M. P., Webster, J. P., Yanez, E., Adams, B., Clark, S., & Lacy, D. (2005). Math in a cultural context: Two case studies of a successful culturally based math project. Anthropology and Education Quarterly, 36(4), 367-385.
Maturana, H. R., & Varela, F. J. (1987). The tree of knowledge: The biological roots of human understanding. Boston: Shambhala.
Miller, J. R. (1996). Shingwauk’s vision: A history of native residential schools. Toronto, ON: University of Toronto Press.
Milloy, J. (1999). A national crime: The Canadian government and the residential school system, 1879-1986. Winnipeg, MB: University of Manitoba Press.
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA: Author.
Native Access to Engineering, (n.d.). Ancestral engineering. Retrieved from http://www.nativeaccess.com/allabout/ancestral.html.
Neel, K. I. S. (2007). Numeracy in Haida Gwaii, BC: Connecting community, pedagogy, and epistemology (pp. 313). Unpublished doctoral dissertation, Simon Fraser University.
Ryan, J. (1992). Aboriginal learning styles: A critical review. Language, Culture, and Curriculum, 5(3), 161-183.
Salkind, N. J. & Rasmussen, K. (2008). Encyclopedia of educational psychology, (Vol. 1). Thousand Oaks, CA: Sage Publications, Inc.
St. Denis, V. (2004). Real Indians: Cultural revitalization and fundamentalism in Aboriginal education. In C. Schick, J. Jaffe, & A. Watkinson (Eds.). Contesting fundamentalisms (pp. 35-47). Halifax, NS: Fernwood.
Statistics Canada. (1996). National Tables: Aboriginal Data. Retrieved from http://www.statcan.ca
Swetz, F. J. (2009). Culture and the development of mathematics: An historical perspective. In B. Greer, S. Mukhopadhyay, A. B. Powell, & S. Nelson-Barber, (Eds.). Culturally responsive mathematics education (pp. 11-42). New York: Routledge.
Wilson, S. (2007). Guest editorial: What is an Indigenist research paradigm? Canadian Journal of Native Education, 30(2), 193-195.